The Treatment of the Line Shift.

The line opacity at a certain sampling frequency $\nu$ is the sum over all (integrated) line opacities $\overline{\chi}_{\rm l}^{}$ multiplied by the line profile function $\varphi_{\rm l}^{}$ ( $\nu$ ):

$\displaystyle \chi_{\rm lb}^{}$ ( $\displaystyle \nu$ ) = $\displaystyle \sum_{\rm lines}^{}$ $\displaystyle \overline{\chi}_{\rm l}^{}$ $\displaystyle \varphi_{\rm l}^{}$ ( $\displaystyle \nu$ ).

(1)

In the static part of the atmosphere a line covers with its Doppler profile ( $\varphi_{\rm D}^{}$ ) only a small frequency range around the transition frequency $\nu_{0}^{}$ (illustrated in Fig. 4 on the right-hand side; note that with respect to our sampling grid, 40 per cent of the available lines are treated in the static part).

Figure 4: Upper panel: the line profile is simply shifted along $\nu_{\rm CMF}^{}$ , represented by the curve. Lower panel: a boxcar profile is assumed for each depth point.

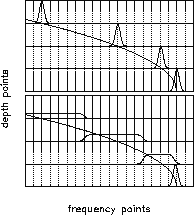
$\begin{figure} \centerline {\hbox{ \psfig{file=10-boxprofile.eps,width=8.5cm,height=8.0cm}}}\end{figure}$

**Figure 4:** *Upper panel:* the line profile is simply shifted along $\nu_{\rm CMF}^{}$ , represented by the curve. *Lower panel:* a boxcar profile is assumed for each depth point.
$\begin{figure} \centerline {\hbox{ \psfig{file=10-boxprofile.eps,width=8.5cm,height=8.0cm}}}\end{figure}$

In the expanding part of the atmosphere the line shift due to the velocity field, $\nu$ = $\nu_{0}^{}$ (1 + v(r)/c), is applied to the individual line opacities before the summation in Eqn. 1 is carried out at each sampling and depth point. However, from the upper panel of Fig. 4 it is obvious that if the line opacity is simply shifted along the comoving frame frequency ( $\nu_{\rm CMF}^{}$ ) to every radius point successively, many frequency points miss the line, since the radius grid is too coarse to treat large line shifts in the observer's frame. This behaviour is corrected by assuming a boxcar profile $\varphi_{\Delta v}^{}$ representing the velocity range around each radius point (see Fig. 4, lower panel).

The convolution ( $\varphi_{\rm D}^{}$ $\otimes$ $\varphi_{\Delta v}^{}$ )( $\nu$ ) results in the final profile function which for $\Delta$ v < v_th gives, as a lower limit, the ordinary opacity sampling; and for sufficiently high velocities ( $\Delta$ v > v_th) the integration over a radius interval represents the Sobolev optical depth ( $\Delta$ $\tau$ ) of a local resonance zone (cf. Sellmaier 1996; Pauldrach et al. 1997). Note that at sufficiently high velocities all lines are included in the radiative transfer if the sampling grid is fine enough. Hence, in this case, the `opacity sampling method' becomes an exact solution.

First results obtained with this procedure have already been published. Sellmaier et al. (1996) showed that their non-LTE line-blocked O-star wind models solve the long-standing HeIIIproblem of HIIregions for the first time. Pauldrach et al. (1996) applied their non-LTE models, based on a slightly different blocking procedure, to SNIa, and Hummel et al. (1997) carried out non-LTE line-blocked models for classical novae.